This is related to the the May 16 post, but takes only the prime indexed terms. Does it still diverge?
Hint
Transform the product into a sum
Hint
The harmonic series 1 + 1/2 + 1/3 + … 1/n +… diverges
I don’t know how to begin proving it, but the more I run this series out, bigger it gets. The conditions of the equation are such that it will always have a consistently non-zero rate of increase, even though that rate of increase decreases each time the formula is cycled ((pn/pn-1) will always be more than (pn+1/pn+1-1), nonetheless any and every (pn/pn-1) will be >1). The divergence will be glacial, but definite.
I can confirm that your intuition for divergence is correct.
I’ve shown that ln(n/n-1) is always larger than 1/n, so Σln(n/n-1) for all natural number n will be larger than the series 1+1/2+1/3+…
but I don’t know how to make sure the sum of all ln(p/p-1) only when p is prime is larger than the provided series
the question is strongly suggesting its divergent, i just dont know how to show it
Perhaps surprisingly, that’s actually good enough since the sum of the prime reciprocals also diverges. However, I’m not letting you just assume that, and proving it is harder than the original problem.
Solution
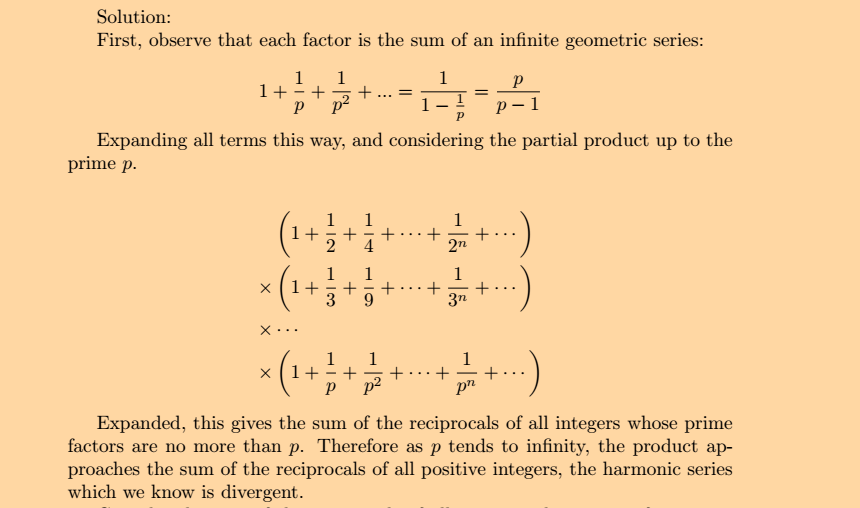
What I like about this solution is that it doubles as a proof that there are infinitely many prime numbers. It’s not circular either – it uses the number theoretic fact that every integer has a prime factorization, but nothing deeper. If only finitely many primes were required for that, … well then of course every finite product converges.