Was wondering about that hint - read it after my solution then tried coming up with another that made the product like (1 + 1/n)^n, but the best I was able to manage was proving that the product is larger than e - an impressive feat since it takes a whopping two terms to get that large… Thought it might be something with writing the product like lim (n → ∞) Π (k = 1 to n) (1 + (n/k)/n), but was never able to figure out a way to do anything with that either.
i added the solution to the post, i didnt see the multiplication before someone mentioned it, but yeah if we put it to the power of e it will telescope again, which is clearly the main character of this sub at this point (jk)
Hint:
spoiler
e
Solution:
spoiler
zkfcfbzr solved it
i put everything into ln because i was scared of multiplication
https://gmtex.siri.sh/fs/1/School/Extra/Maths/Qotd solutions/2024-05-16_telescoping-multiplication.html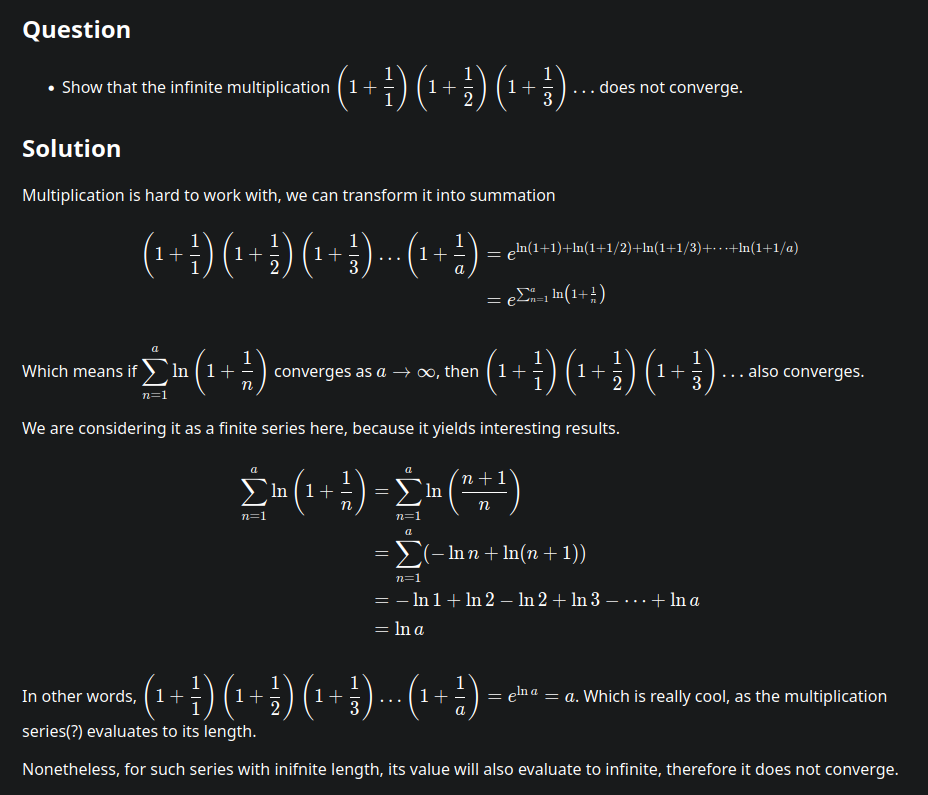
comment
Was wondering about that hint - read it after my solution then tried coming up with another that made the product like (1 + 1/n)^n, but the best I was able to manage was proving that the product is larger than e - an impressive feat since it takes a whopping two terms to get that large… Thought it might be something with writing the product like lim (n → ∞) Π (k = 1 to n) (1 + (n/k)/n), but was never able to figure out a way to do anything with that either.
i added the solution to the post, i didnt see the multiplication before someone mentioned it, but yeah if we put it to the power of e it will telescope again, which is clearly the main character of this sub at this point (jk)